本文最后更新于 945 天前,其中的信息可能已经有所发展或是发生改变。
队列
队列(Queue)是一个数据集合,仅允许在列表的一端插入,在另一端删除。进行插入的一端称为“队尾”(rear),插入的动作称为入队;进行删除的一端称为“队头”(front),删除的动作称为出队。队列的性质是先进先出(FIFO,First-in-First-out)。下图为一个例子:
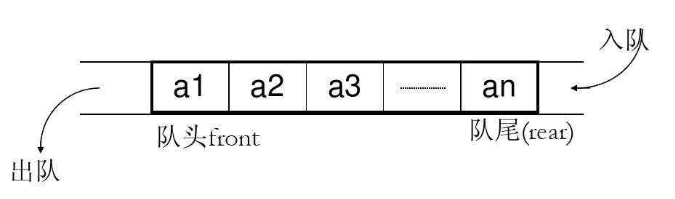
队列的实现方式:环形队列
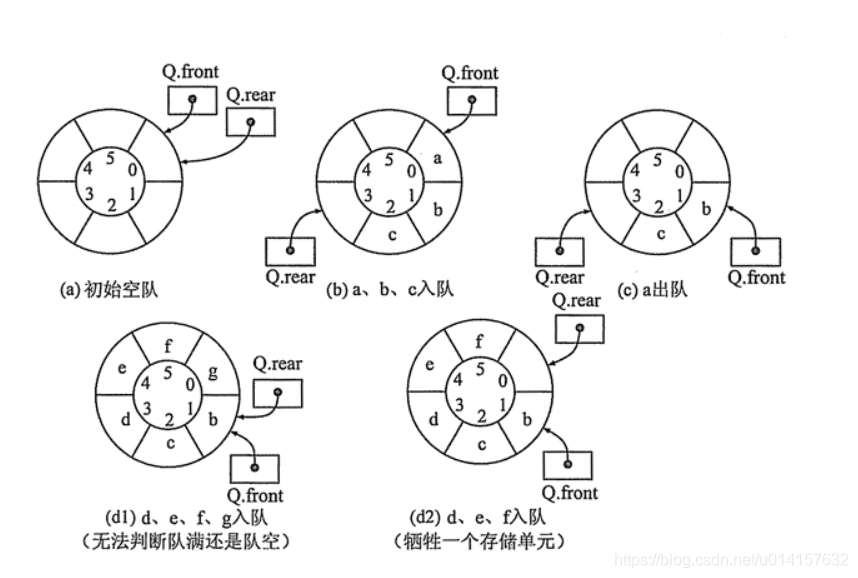
设队列的最大长度为maxsize,队头指针为front,队尾指针为rear。上图中,队列最大长度为5,初始空队列front和rear都指向0。
- 当
front == rear时,队为空,如图(a) - 当
(rear + 1) % maxsize == 0,队满。对于图(d1)的情况front == rear,无法判断到底是队空还是队满,因此需要牺牲一个存储单元,如图(d2)。 - 出队时队头指针前进1,
front = (front + 1) % maxsize。 - 入队时队尾指针前进1,
tear = (tear + 1) % maxsize。
上面的式子中,都有对maxsize的取余,因为比如当指针达到最大值5时,再加1就会超过5,此时取余可以重新进入队列循环。
栈
栈(stack)是一个数据集合,只能在一端进行插入和删除,其特点是后进先出(LIFO,lats-in-first-out)。栈的节本操作有进栈(push)、出栈(pop)、取栈顶(gettop)。其示意图如下所示:

对于python来说,使用列表即可实现栈。设列表为stack,其三个基本操作为:
- 入栈:
stack.append() - 出栈:
stack.pop() - 取栈顶:
stack[-1]
例题
有效的括号
此题为leetcode第20题思路:使用栈,从给定的括号字符串第一个开始,依次往后,左半边括号入栈,遇到与之对应的右边吧括号出栈,直到最后若栈为空,则为有效表达,否则为无效表达。代码如下:
class Solution:
def isValid(self, s: str) -> bool:
map = {')':'(', ']':'[', '}':'{'}
stack = []
for char in s:
# 如果是右括号
if char in map:
top = stack.pop() if stack else '#'
if map[char] != top:
return False
# 如果是左括号
else:
stack.append(char)
return not stack用队列实现栈
此题为leetcode第225题思路:使用两个队列可以实现栈,对于栈的push操作,可以由两个队列实现:
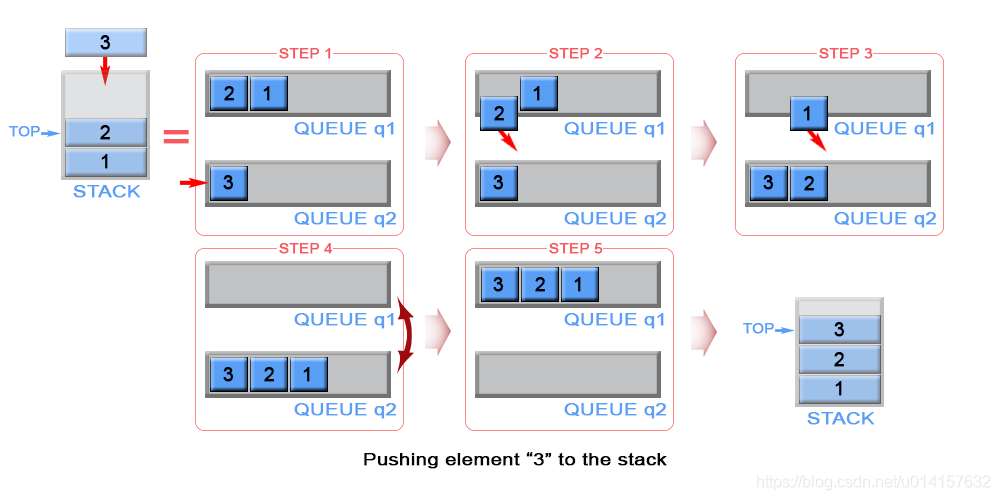
对于pop操作,直接从q1弹出即可
from collections import deque
class MyStack:
def __init__(self):
'''
Initialize your data structure here.
'''
self.q1 = deque()
self.q2 = deque()
def push(self, x: int) -> None:
'''
Push element x onto stack.
'''
self.q2.append(x)
while self.q1:
self.q2.append(self.q1.popleft())
self.q1, self.q2 = self.q2, self.q1
def pop(self) -> int:
'''
Removes the element on top of the stack and returns that element.
'''
return self.q1.popleft()
def top(self) -> int:
'''
Get the top element.
'''
return self.q1[0]
def empty(self) -> bool:
'''
Returns whether the stack is empty.
'''
return len(self.q1) == 0用栈实现队列
此题为leetcode第232题思路:同样可以用两个栈实现队列,对于push操作:
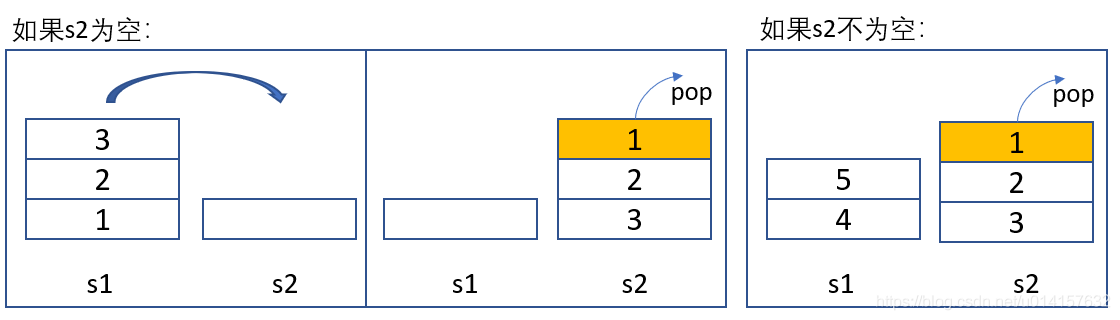
class MyQueue:
def __init__(self):
'''
Initialize your data structure here.
'''
self.s1, self.s2 = [], []
def push(self, x: int) -> None:
'''
Push element x to the back of queue.
'''
while self.s1:
self.s2.append(self.s1.pop())
self.s1.append(x)
while self.s2:
self.s1.append(self.s2.pop())
def pop(self) -> int:
'''
Removes the element from in front of queue and returns that element.
'''
return self.s1.pop()
def peek(self) -> int:
'''
Get the front element.
'''
return self.s1[-1]
def empty(self) -> bool:
'''
Returns whether the queue is empty.
'''
return len(self.s1) == 0